The Chain Rule, Part 2

Misc

I’m out of town tomorrow and Friday.

There’s an online discussion aimed at exploring some applications of derivatives that we’ll do in lieu of class meetings those days.

Questions?

The Chain Rule

Multiple Uses

Find dy/dx if y = sin²(x³ - 7x).

There are really 3 functions nested inside of each other here: squaring, applied to sine, which in turn is applied to x³ - 7x. So you solve this by using the chain rule twice, once on sin², and then again on sin(x³ - 7x):

In more detail, what we did here was

• Use the chain rule with squaring as the outer function and sin(x³ - 7x) as the inner function.
• Use the power rule to differentiate the square of something.
• Now the chain rule requires multiplying by the derivative of sin(x³ - 7x).
• Use the chain rule a second time to find that derivative, with sine as the outer function and x³ - 7x as the inner.
• Differentiate sine to cosine.
• The chain rule requires multiplying by the derivative of x³ - 7x.
• Use the power, difference, and constant multiple rules to find this derivative.

The Chain Rule in dy/dx Notation

Find dy/dx if y = (x²+1) cos(x²+1).

Since x²+1 appears twice here, it is tempting to give it a name, say u, and then simplify the problem by writing y as u cos u.

Now differentiating with respect to u is just a use of the product rule...

... except you also have to remember that we don’t really want dy/du, we want dy/dx, and u is a function (specifically, the inner function for purposes of the chain rule) of x. So we have to use the chain rule, i.e., multiply by du/dx.

So what we did was calculate dy/dx as dy/du times du/dx. If that was a product of ordinary fractions, we’d explain it by saying the “du” in a denominator canceled out the “du” in a numerator. While this isn’t quite what happened (du isn’t really a number), it’s a fine alternative way to remember the chain rule.

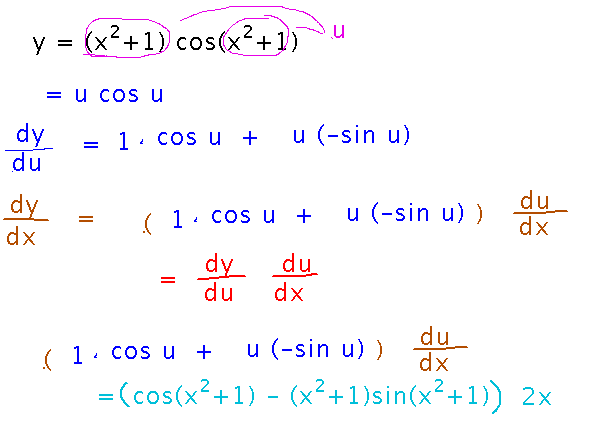
In detail, what we did here was...

• Temporarily treat the inner function, x² + 1, as a variable, u.
• Calculate dy/du, using the product rule.
• Multiply the result by du/dx, calculating du/dx via the power rule.
• Rewrite any leftover u terms as x² + 1.

Next

Implicit differentiation.

Read section 3.7 for Monday.