SUNY Geneseo Department of Mathematics

The Squeeze Theorem

Wednesday, February 20

Math 221 03
Spring 2019
Prof. Doug Baldwin

Return to Course Outline

Previous Lecture

Misc

SI this afternoon. 3:00, Bailey 209.

Questions?

The Squeeze Theorem

Last part of section 2.3.

Example

(From book)

Find limx→0 x2 sin(1/x)

We first used this Mathematica notebook to look at some plots of the function in question. Notice how it oscillates ever faster as x approaches 0, but with ever decreasing frequency.

Now the key realization is that sin(1/x) is always between -1 and 1, and so when you multiply it by x2 you get a complicated-looking function but with simple bounds on its values:

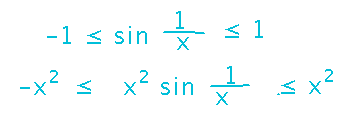
sin(1/x) is between -1 and 1, so x^2 sin(1/x) is between -x^2 and x^2

Now we can take limits of all the functions involved, and by the squeeze theorem know that the limit of the function in question is actually the limits of the two simple bound functions:

Limits of -x^2 and x^2 are both 0, so is limit of x^2 sin(1/x) since it lies between those other limits

Next

Apply the squeeze theorem to derivatives of trigonometric functions.

Read section 3.5, and while doing so try to identify the parts that would be relevant to deriving the derivative of cos x. We’ll start tomorrow’s class by doing that.