Limit Laws

Return to Course Outline

Previous Lecture

Misc

Thank you to people who have made appointments to grade the problem set.

Demonstration of how to make appointments with Google calendar.

Also see this tutorial on Google calendar appointments by CIT:

Questions?

Laws for Arithmetic with Limits

“Evaluating Limits with the Limit Laws” and “Limits of Polynomial and Rational Functions” subsections of section 2.3.

Examples

Find lim_x→1 2x + 3

What ideas from the reading seem helpful here?

The sum law, the constant multiple law. Generally, the collection of laws that let you move limits “inside” arithmetic operations.

Finding the limit...

Apply limit laws one by one until the problem is reduced to arithmetic on numbers:

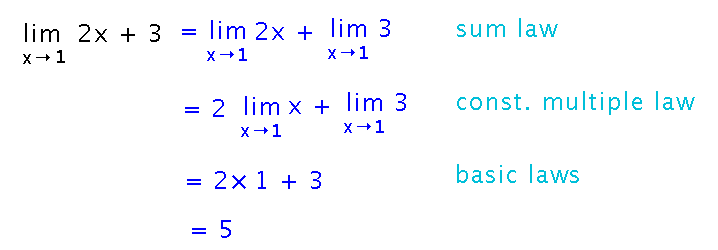
Notice that you can get the same result by simply plugging x = 1 into the function, 2x + 3. That you can do this (as long as the function is defined at that x value) is a consequence of the fact that there is a limit law for each arithmetic operation, and that all the laws basically say you can move the limit inside the operation. This provides a very helpful shortcut for evaluating simple limits.

Find lim_x→0 (x+1)² / √(x+2)

Similar to the example above, you can do this by step-by-step application of limit laws. Or, once you verify that there is a limit law for each operation used here, you can take the shortcut of plugging x = 0 into the function:

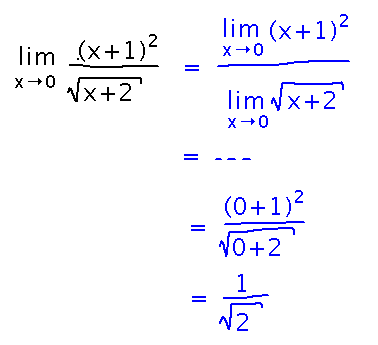
Where Do the Laws Come From?

They all come from the definition of limit. For example, the basic law lim_x→a x = a roughly comes from asking whether you can always keep x (the function) within tolerance ε of a (the limit), and realizing that you can by keeping x (now remembered as also being the input to the function) within that same tolerance of a (now used as the value x is approaching).

Key Points

Limits can be moved inside arithmetic operations (as long as the result is defined).

There are reasons you can do this, all traceable back to the definition of “limit.”

Next

Using algebraic simplifications to find limits.

Example: find lim_x→2 (x² - x - 2) / (x-2)

We can’t use limit laws on this, because when x = 2, the denominator in the fraction is 0, and so the quotient law doesn’t apply (and if you don’t notice that, the whole fraction becomes 0/0 when x = 2, which should tell you that something is wrong).

But you can factor the numerator and cancel the denominator, leaving something you can use limit laws on:

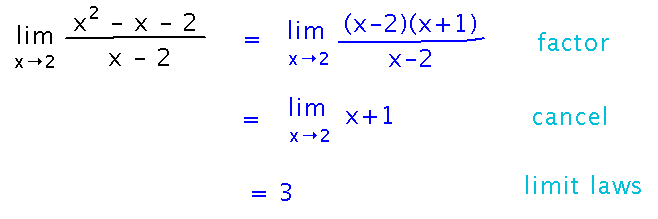
Technical point: (x² - x - 2) / (x-2) and x + 1 are not the same function, even though one simplifies to the other. The reason is that for 2 functions to be the same, they must have the same values and the same domain, and the domain of (x² - x - 2) / (x-2) is missing one point compared to the domain of x+1:

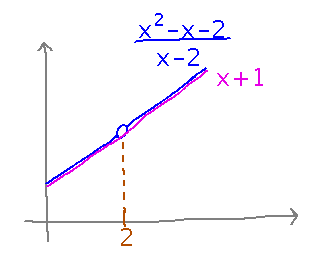
So why can you use the limit of one function to find a limit of a different one? Again, this is a consequence of the definition of limit: if the functions are equal everywhere except maybe at one point, then any ε and δ that “work” to prove that one of the functions has a certain limit at that point will also work to prove that the other function has the same limit (since δ can’t be 0, the definition of limit never cares that the functions differ at the point).

Read the “Additional Limit Evaluation Techniques” subsection of section 2.3, up to but not including the place where one-sided limits appear. (In other words, read from the middle of page 163 through the top 2/3 of page 167.)

Next Lecture