SUNY Geneseo Department of Mathematics
Friday, December 8
Math 221 05
Fall 2017
Prof. Doug Baldwin
Tuesday, Dec. 12, 9:30 - 10:30 AM, Fraser 213 (our Thursday room).
You bring topics/questions you want to review.
Thursday, December 14, 8:00 AM, in our MWF Sturges classroom (Sturges 223).
The exam will cover the whole semester, but emphasizing material since the second hour exam (e.g., optimization; the Fundamental Theorem; substitution; area, volume, and similar applications of definite integrals; etc.)
Roughly 2 to 2 1/2 times as long as the hour exams in terms of number of questions and design time. But note that you have roughly 4 times as long in terms of actual time, so there should be less time pressure.
The rules and format will otherwise be the same as on the hour exams, particularly the open-references and calculator rules.
I’ll bring donuts and cider.
Available from now through December 12.
Please fill them out — they’re useful to me in improving courses over time, especially written comments.
Get to the SOFI survey via Knightweb.
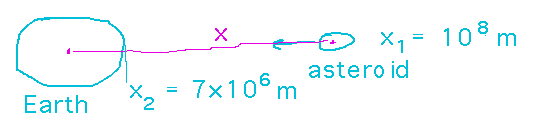
(Or why you shouldn’t drop asteroids on your planet)
Imagine an asteroid dropping towards Earth under the force of gravity. The main variable here is x, distance from the center of Earth, measured in meters (this asteroid is actually not dropping from very far away, only about 1/4 to 1/3 the distance to the moon).

The work done by gravity on the asteroid becomes its energy when it reaches Earth, so calculate what that work is. The reading gave a formula for the work done by a force, namely the integral of that force over the distance over which it acts:

Physics provides equations for gravitational force as a function of distance. We also have values for the gravitational constant and the masses of Earth and an asteroid of around the size that wiped out the dinosaurs (G is about 7×10-11 N m2/kg2, mass of Earth is about 6×1024 kg, reasonable asteroid mass is about 1016 kg).
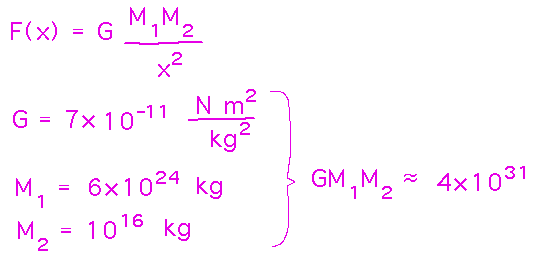
Factoring the constants out of the work integral gives

Which integrates using the power rule for antiderivatives (remembering that you add 1 to the exponent even with negative exponents).
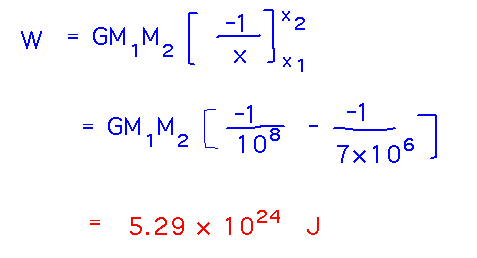
The final work done by gravity on the asteroid is around 5 × 1024 Joules. It’s all released when the asteroid hits Earth. By way of comparison, the atom bombs that destroyed cities in World War 2 released around 1013 Joules, about 100 billion times less. So no wonder there aren’t any dinosaurs left, and we’d rather not have another asteroid hit Earth any time soon.
The work done by a varying force can be modeled as the integral of that force with respect to distance moved.
So to find work given a formula for force and starting and ending distances, integrate the force from the starting to the ending distance.
Work in the context of pumping fluids.