- Colloquium
- Zubair Dawood, Manning & Napier
- “Mathematical Methods in Equity Research”
- Thursday, February 19, 3:00 - 4:00
- Newton 203
- Extra credit for summaries
- Section 8.4
- Substitute trigonometric expression for x
- Use derivative of that expression for dx
- May imply restrictions on x
- Reference triangle helps with reverse substitution
- Examples
- Integrate x / √(1-x2)
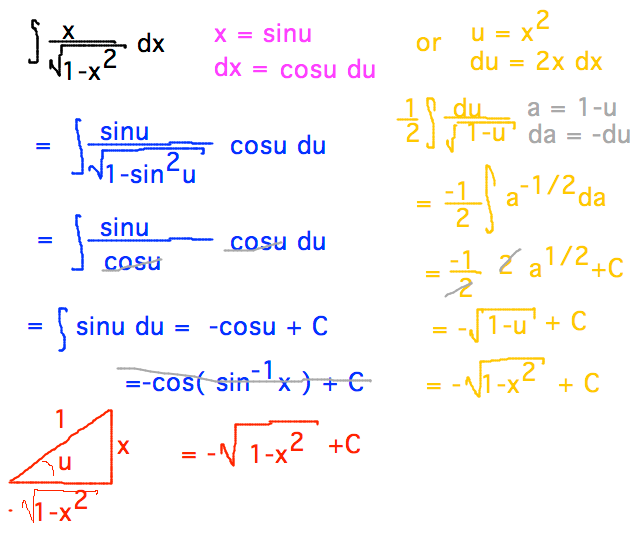
- General pattern is identify trig function that could be inserted for x, insert derivative for dx, use trig identities and algebra to simplify into integrable form, and finally rewrite u (especially in trig functions) using reference triangle
- Trig substitutions often overlap with other methods of integration, as this one did with classical u substitution
- Integrate √(1-x2)

- Trig substitutions are a little more flexible than u substitutions, because you can replace x with any function you like and dx with its derivative, you don’t have to have a pre-existing function-and-derivative relation between parts of the integrand like in u substitution
- But down side is that the set of substitutions that are likely to be useful with trig substitution is smaller than the ones that might be useful in u substitution
- Could you also use the substitution x = cosu?

- Yes, and it yields the same answer, except for a constant that can be absorbed into the “+ C”
- Integrate x / √(1-x2)
- Partial Fractions
- Read section 8.5