Integrating Trigonometric Forms

Return to List of Lectures

Previous Lecture

• Questions?
• Trigonometric Integration
  • Section 8.3
    • Identities relating sin-cos, sec-tan help evaluate integrals
      • tan²x = sec²x - 1
      • cot²x = csc² - 1
      • sin²x = 1 - cos²x
  • Examples
    • Integrate sin²x cos³x
      
      • Notice that the goal is not to completely rewrite one of the functions in terms of the other, it’s to leave one instance alone to act as the “du” in a substitution
    • Integrate sin3x cos4x
      
      • Relevant identity here is a generalization of double angle and angle sum rules for sine and cosine; see textbook page 461
    • Integrate sec⁴x tan³x
      
      • Key idea is to break up the sec⁴x into part that can be rewritten in terms of tanx and part that is the derivative of tanx for substitution.
      • Coming up with decomposition is a matter of looking for parts you know how to integrate and other parts from which you can get either easy-to-integrate terms or their derivatives for a later substitution
• Next
  • Trigonometric substitution
    • Read section 8.4
  • Substitution in general
    • Variables are unknowns, so you substitute for them (as long as you carefully follow through all the implications of such substitutions, e.g., for derivatives appearing in a formula)
    • Example: substitution to give you a different way of looking at a function
      
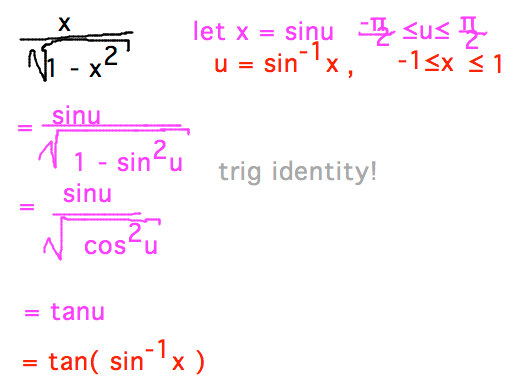
    • Trigonometric substitutions use this idea to rephrase integrands into easily-integrated forms

Next Lecture