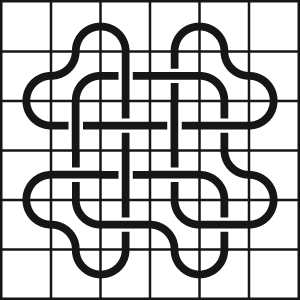
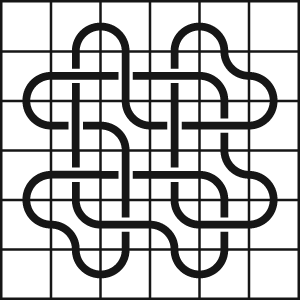
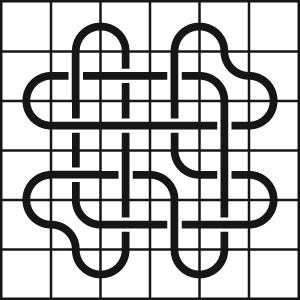
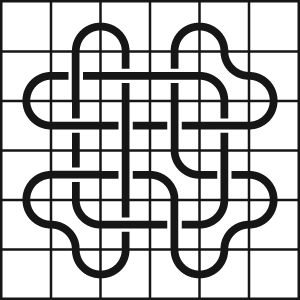
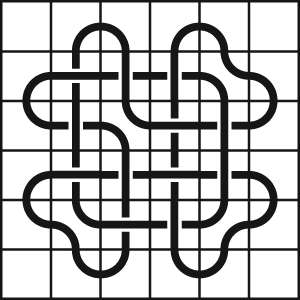
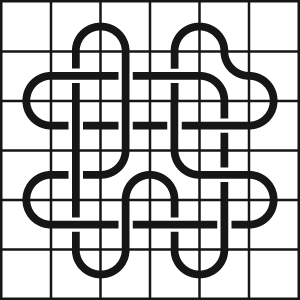
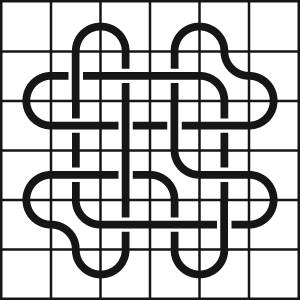
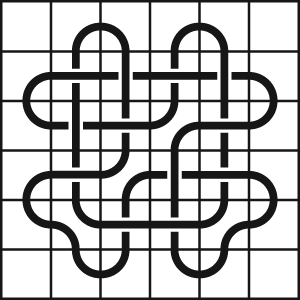
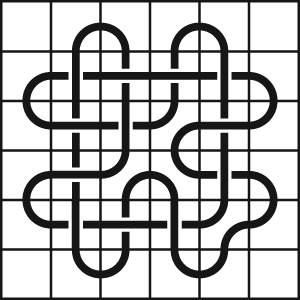
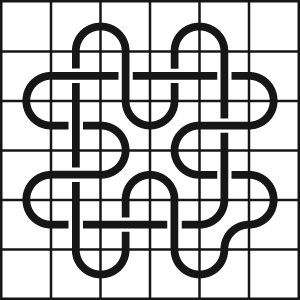
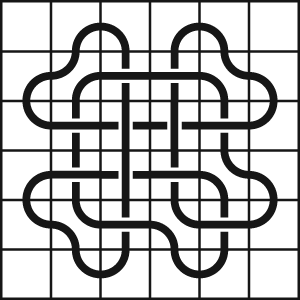
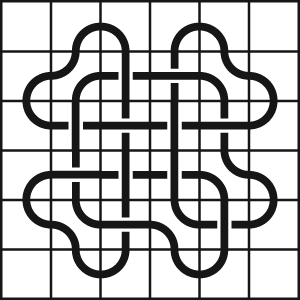
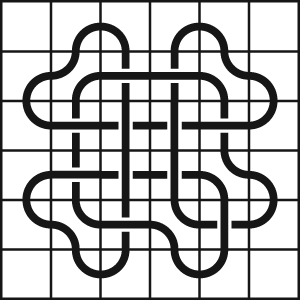
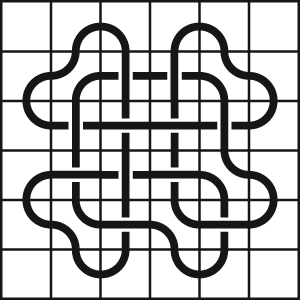
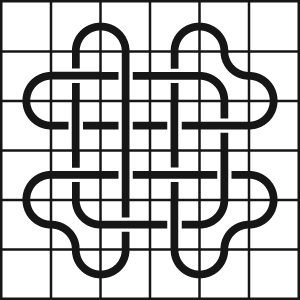
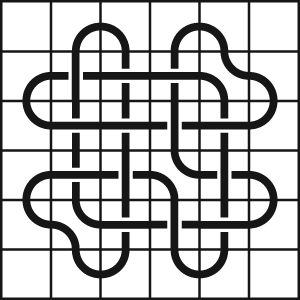
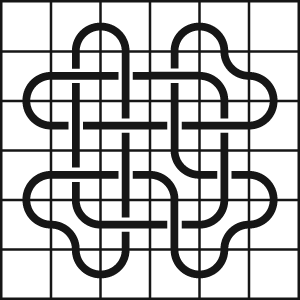
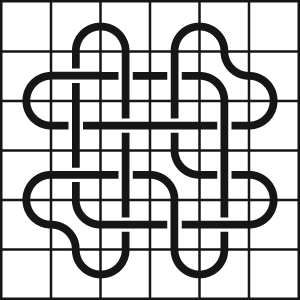
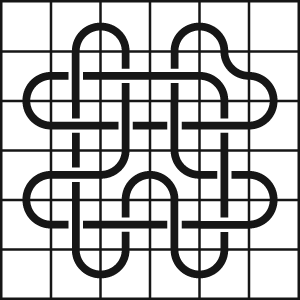
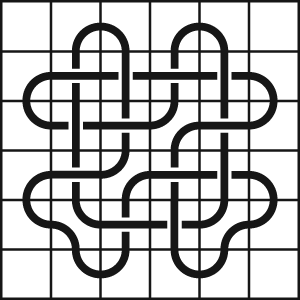
Table of Knot Mosaics with Mosaic Number 6 or Less
Mosaic number 5 or less			Mosaic number 6 or less			Mosaic number 7
Crossing number:	10 or less	11	12	13	14	15	16
(Click mosaic for larger view.)
When listing prime knots with crossing number 10 or less, we will use the Alexander-Briggs notation, matching Rolfsen’s table of knots. [Rolfsen]
		*These knots are listed as 10162‑10166 in Rolfsen due to the Perko Pair.
When listing prime knots with crossing number 11 or more, we use the Dowker-Thistlethwaite name of the knot. See KnotInfo for more information.
All knots with mosaic number 6 and crossing number 11:
All knots with mosaic number 6 and crossing number 12:
All knots with mosaic number 6 and crossing number 13:

References:

Heap, A.; Knowles, D. Tile Number and Space-Efficient Knot Mosaics; J. Knot Theory Ramif. 2018, 27.
Heap, A.; Knowles, D. Space-Efficient Knot Mosaics for Prime Knots with Mosaic Number 6; Involve 2019, 12.
Heap, A.; LaCourt, N. Space-Efficient Prime Knot 7-Mosaics; Symmetry 2020, 12.
Heap, A.; Baldwin, D.; Canning, J.; Vinal, G. Knot Mosaics For Prime Knots with Crossing Number 10 or Less; in preparation.
Kuriya, T.; Shehab, O. The Lomonaco–Kauffman Conjecture; J. Knot Theory Ramif. 2014, 23.
Lee, H.; Ludwig, L.; Paat, J.; Peiffer, A. Knot Mosaic Tabulation; Involve 2018, 11.
Lomonaco, S.J.; Kauffman, L.H. Quantum Knots and Mosaics; Quantum Inf. Process. 2008, 7, 85–115.
Ludwig, L.; Evans, E. An Infinite Family of Knots Whose Mosaic Number Is Realized in Non-reduce Projections; J. Knot Theory Ramif. 2013, 22.
Rolfsen, D. Knots and Links; Publish or Perish Press: Berkeley, CA, USA, 1976.

[Back to Top]