Partial Derivatives

Return to Course Outline

Previous Lecture

Anything You Want to Talk About?

(No.)

Partial Derivatives

Based on “Derivatives of a Function of Two Variables” and “Functions of More than Two Variables” in section 3.3.

Key Ideas

The limit definition of a partial derivative (see the book) is the theoretical foundation for partial derivatives.

To find a partial derivative with respect to, say, x, treat everything except x as a constant. This is important as a practical rule for evaluating partial derivatives.

A partial derivative is the slope of a tangent line, but in one dimension; partial derivatives can be estimated by slopes of secant lines in one dimension.

Partial derivatives apply to functions with any number of dimensions/variables.

Questions

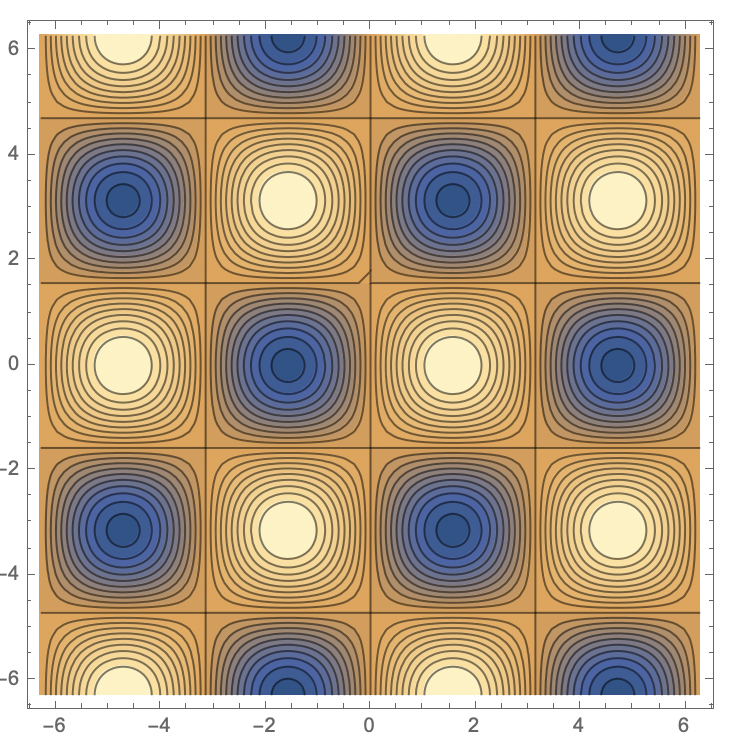
An example from a contour map?

Here’s an example of a contour plot. It’s probably most useful for visually comparing partial derivatives; for example places where contour lines are closer together as you move horizontally or vertically are places where partial derivatives have higher magnitudes. You can also make numerical estimates if you know the function values associated with adjacent contour lines and the x and y values for horizontally or vertically separated points on those contours.

Examples

Find the partial derivatives with respect to x and y of f(x,y) = x² + xy + y².

This is mostly an exercise in what “treat the other variables as constant” means in practice.

Find the partial derivatives with respect to u, v, and w of √(u² + v² + w²).

Here there are 3 variables, although because of the symmetry in how they’re used in the function, all 3 derivatives work in the same way. Finding them involves using the chain rule within each partial derivative (a good illustration of how single-variable differentiation rules can all be used while finding partial derivatives).

Imagine a hillside that comes with a handy XY coordinate system, so that the height of the hill can be described by the equation h(x,y) = (2-y) sin(πx). If you’re standing at point (1,1), does your elevation change faster if you move in the positive x direction, or if you move in the positive y direction?

Find the partial derivatives of h, and then compare their values at point (1,1). Whichever has the higher magnitude corresponds to the direction in which elevation changes fastest.

Partial Derivatives in Mathematica

Use the D function, and specify which variable you want to differentiate with respect to. (This is a big part of the reason D takes the variable as an argument in the first place.)

Download this notebook for some examples (and plots of the “egg carton” function that we used for the partial derivatives and contour plots discussion).

Next

Higher-order partial derivatives.

Please read “Higher-Order Partial Derivatives” in section 3.3 of the textbook.

Have a good break!

Next Lecture